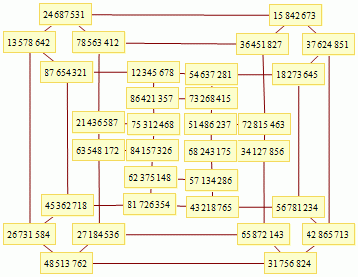
When a deck of cards is shuffled, the description of how the card positions changed is called a permutation. A set of permutations can generate a group, an extrememly valuable math concept for understanding symmetries. The map of orderings reachable by a set of permutations is called a Cayley graph. For 8 cards, the Hindu shuffle on 12345678 gives 87654321, and the Mongean shuffle on 12345678 gives 86421357. The Mongean shuffle on 54637281 gives 12345678. The Cayley graph below shows all 32 arrangements that 8 cards can get with these two perfect shuffles. With imperfect shuffles, any arrangement of cards is eventually possible. A side effect of perfect shuffling is that it allows incredible card control.